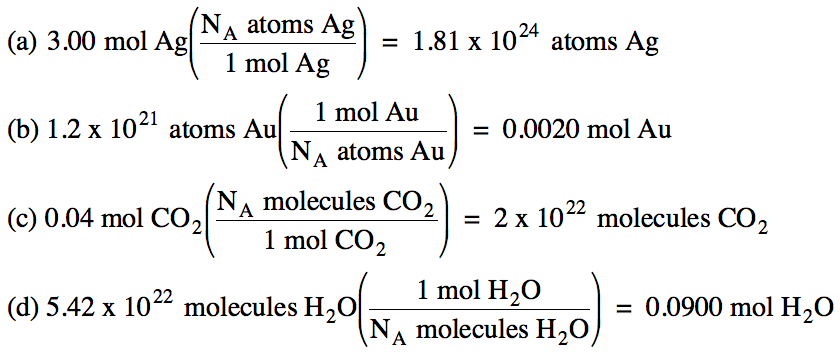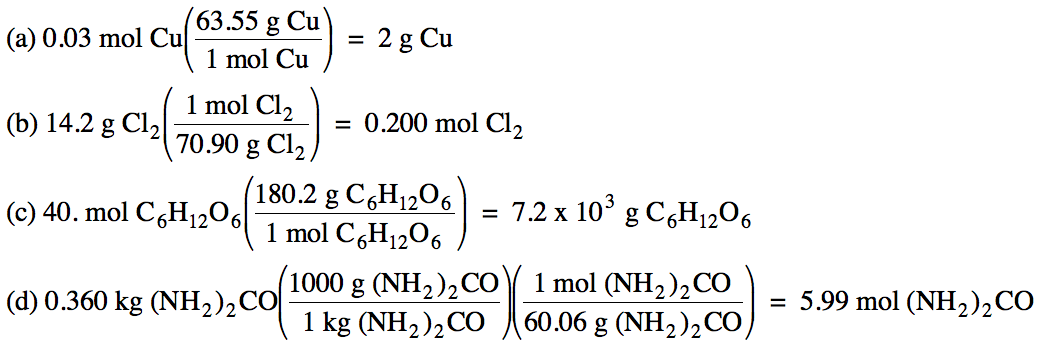Chapter 2: The Mole
Section 2-1: Introduction to Avogadro's Number and the Mole
Section 2-3: Converting Between Mass and Number of Atoms or Molecules
Chapter 2 Practice Exercises and Review Quizzes
Section 2-1: Introduction to Avogadro's Number and
the Mole
The unit mole (mol) is used to describe the amount of a substance:
1 mol =
6.02 x 1023 atoms or molecules (more than one bonded atom)
The number 6.02 x 1023
is known as Avogadro's number and
will be given the symbol NA in calculations throughout this
textbook. The following problem
will demonstrate how to convert back and forth between an amount in moles and
the number of atoms or molecules using dimensional analysis:
Sample Exercise 2A:
Convert the following:
(a) 3.00 moles of silver to number
of silver atoms
(b) 1.2 x 1021 gold
atoms to moles of gold
(c) 0.04 moles of carbon dioxide to
number of carbon dioxide molecules
(d) 5.42 x 1022 water
molecules to moles of water
Solution:
Section 2-2: Molar Mass
The mass of one mole of an element
or compound in the unit grams per mole (g/mol) is known as the molar mass (M) and can be obtained from the periodic table. Note that the molar masses found on
different periodic tables may differ slightly. Throughout this textbook, we will round all molar mass
values to 4 sig. fig.s. The following table shows some examples:
|
Element or Compound |
M (g/mol) |
|
Cu |
63.55 |
|
Cl2 |
2(35.45)
= 70.90 |
|
C6H12O6 |
6(12.01)
+ 12 (1.008) + 6(16.00) = 180.2 |
|
(NH2)2CO |
2(14.01)
+ 4(1.008) + 12.01 + 16.00 = 60.06 |
Once we have obtained the molar
mass of an element or compound, we can convert back and forth between an amount
in moles and the mass using dimensional analysis as demonstrated in the
following problem:
Sample Exercise 2B:
Convert the following:
(a) 0.03 moles of copper to grams
(b) 14.2 grams of Cl2 to
moles
(c) 40. moles
of C6H12O6 to grams
(d) 0.360 kilograms of (NH2)2CO to moles
Solution:
Section 2-3: Converting Between Mass and Number of Atoms or
Molecules
We can combine the methods used in
the previous two sections to convert back and forth between mass and the number
of atoms or molecules using dimensional analysis and one of the following
strategies:
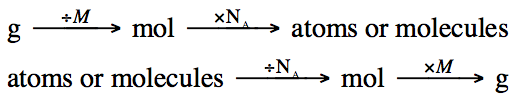
The following problem will demonstrate
each of the strategies above:
Sample Exercise 2C:
Convert the following:
(a) 0.080 grams of I2 to
number of I2 molecules
(b) 2.29 x 1024 CS2
molecules to kilograms of CS2
Solution:
(a) M of I2 = 2(126.9) = 253.8 g/mol
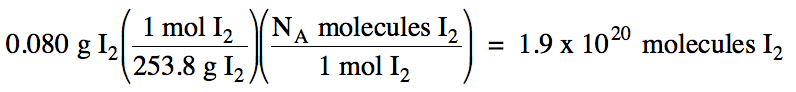
(b) M of CS2 = 12.01 + 2(32.07) = 76.15 g/mol
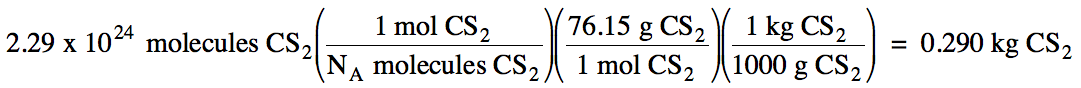
Note that it was not necessary to
stop and actually calculate the moles along the way to finding the final
answer.
If we know the number of molecules
in a sample, we can calculate the number of atoms of any element present in the
sample as follows:
Sample Exercise 2D:
A sample contains 8.40 x 1025 molecules C6H4(NO2)2. How many oxygen atoms are in the sample?
Solution:
We see from the chemical formula
that one molecule contains 4 oxygen atoms, so we simply multiply the number of
molecules by a conversion factor representing the number of oxygen atoms per
one molecule:
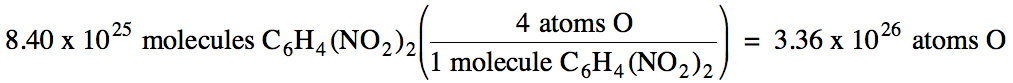
Chapter 2 Practice Exercises and Review Quizzes:
2-1) Convert the following:
a. 20. kilograms iron to number of iron
atoms
b. 2.76 x 1017 zinc
atoms to milligrams zinc
Click for Solution
2-1)
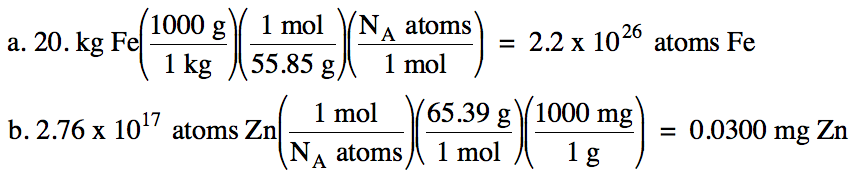
2-2) The density of solid C6H4(OH)2 is 1.3 g/cm3. What is
the volume of 4.45 x 1023 C6H4(OH)2 molecules?
Click for Solution
2-2) M of C6H4(OH)2
= 6(12.01) + 6(1.008) + 2(16.00) = 110.1 g/mol
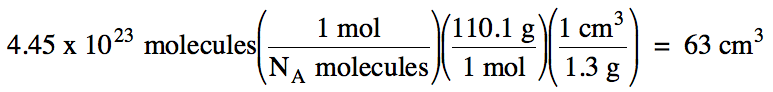
2-3) The density of liquid (CH3)3N
is 0.670 g/mL. How many molecules
are in 9.6 liters of (CH3)3N? How many hydrogen atoms are in this sample?
Click for Solution
2-3) M of (CH3)3N =
3(12.01) + 9(1.008) + 14.01 = 59.11 g/mol